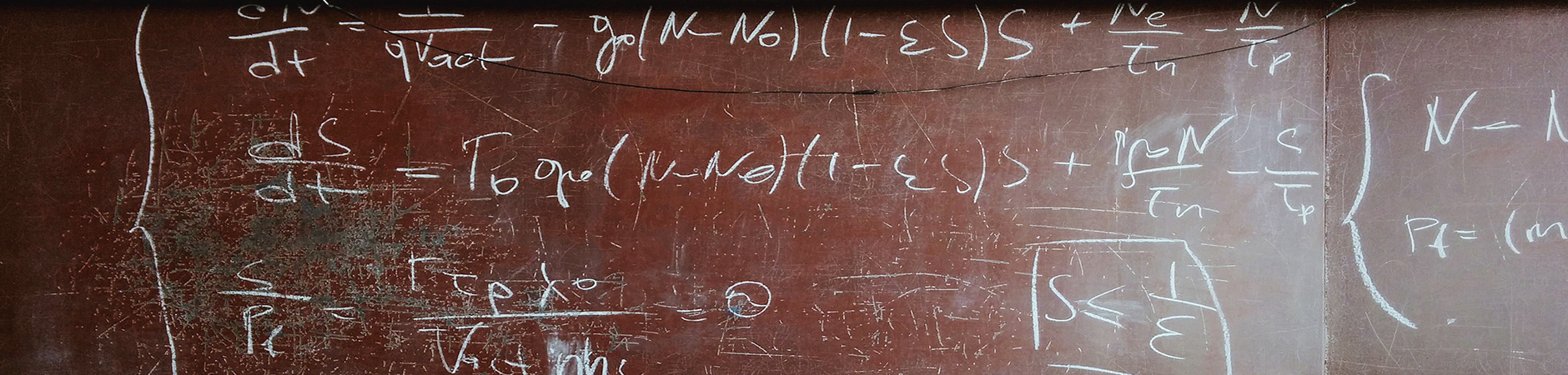
Research in Mathematical Physics in Heidelberg takes place at a variety of interfaces between the two disciplines, ranging from the analysis of functional renormalization and statistical mechanics to applications of ideas from quantum field theory in topology and algebraic geometry. We aim both to develop mathematical theories as required and inspired by physical considerations, and to achieve mathematically rigorous treatments of relevant physical phenomena.
The fruitful interaction of mathematics and physics is at the very heart of the cluster of excellence STRUCTURES at Heidelberg University.
Many Body Physics: The activities center on many-body theory, quantum field theory and statistical mechanics. A main interest is the mathematical construction of correlated-fermion models by multi-scale methods, with applications in the theory of unconventional superconductivity and other symmetry-broken phases of matter.
Random Tensors and Field Theory: The activities center on many-body theory, quantum field theory and statistical mechanics. A main interest is the mathematical construction of correlated-fermion models by multi-scale methods, with applications in the theory of unconventional superconductivity and other symmetry-broken phases of matter. (Group: Prof. Razvan Gurau)
The activities derive from the interaction between geometry and high-energy physics that have arisen in string theory since the 1980's. Research builds on supersymmetric and topological field theories, and supergravity. A central topic is mirror symmetry in its various formulations, and the mathematical theory of BPS invariants.
Another central theme is the geometry underlying the so-called String Theory Landscape -- an extremely large set of solutions, one of which possibly describes our universe.